Types of Price Elasticity of Demand | Free Economic Blogs
Types of Price Elasticity of Demand

Price Elasticity of Demand
According to Prof. Alfred Marshall, price elasticity of demand is a ratio of proportionate change in the quantity demanded of a commodity to a given proportionate change in its price.
Ed = Percentage change in Quantity Demanded / Percentage change in Price
Symbolically,
Ed = %∆Q / %∆P ,
Ed = ∆Q/Q ÷ ∆P/P
Ed = ∆Q/Q × P/∆P
Where,
Q = Original quantity demanded
∆Q = Difference between the new quantity and original quantity demanded
P = Original price
∆P = Difference between new price and original price
Following are the types of Price Elasticity of Demand:
1) Perfectly Elastic Demand (Ed = ∞) :
When a slight or zero change in the price brings about an infinite change in the quantity demanded of that commodity, it is called perfectly elastic demand. It is only a theoretical concept. For example, a 10% fall in price may lead to an infinite rise in demand.
Ed = Percentage change in Quantity Demanded / Percentage change in Price = ∞
Ed = ∞
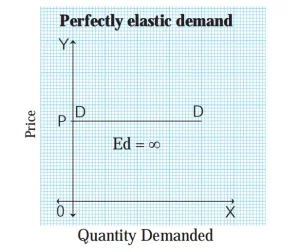
In the above figure, the demand curve DD is a horizontal line parallel to the X-axis indicating perfectly elastic demand.
2) Perfectly inelastic demand (Ed = 0) :
When a percentage change in price has no effect on the quantity demanded of a commodity it is called perfectly inelastic demand. For example, a 20% fall in price will have no effect on the quantity demanded.
Ed = %∆Q / %∆P
Ed = 0/20 = 0
Ed = 0
In practice, such a situation rarely occurs. For example, demand for salt, and milk.

In the above figure, when price rises from OP to OP1 or when the price falls from OP to OP2, demand remains unchanged at OQ. Therefore, the demand curve is a vertical straight line parallel to the Y axis, indicating perfectly inelastic demand.
3) Unitary elastic demand (Ed = 1) :
When a percentage change in price leads to a proportionate change in quantity demanded then demand is said to be unitary elastic. For example, 50% fall in price of a commodity leads to 50% rise in quantity demanded.
Ed = %∆Q / %∆P
Ed = 50/50 = 1
∴ Ed = 1
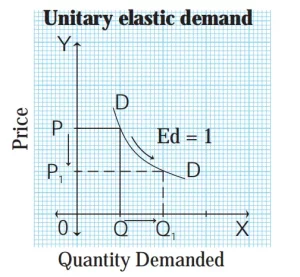
In the above figure, when price falls from OP to OP1 (50%), demand rises from OQ to OQ1 (50%). Therefore, the slope of the demand curve is a ‘rectangular hyperbola’.
4) Relatively elastic demand (Ed >1) :
When a percentage change in price leads to more than proportionate change in quantity demanded, the demand is said to be relatively elastic. For example, 50% fall in price leads to 100% rise in quantity demanded.
Ed = %Q / %P
Ed = 100/50
∴Ed = 2
Ed > 1
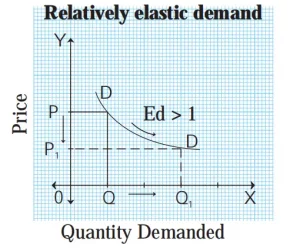
In the above figure, when price falls from OP to OP1 (50%), demand rises from OQ to OQ1 (100%). Therefore, the demand curve has a flatter slope.
5) Relatively inelastic demand (Ed < 1) :
When a percentage change in price leads to less than proportionate change in the quantity demanded, demand is said to be relatively inelastic. For example, 50% fall in price leads to 25% rise in quantity demanded.
Ed = %∆Q / %∆P = 25/50 = 0.5
Ed = 0.5
∴Ed < 1

In the above figure, when price falls from OP to OP1 (50%), demand rises from OQ to OQ1 (25%). Therefore, the demand curve has a steeper slop


